If AD and PM are medians of triangle ABC and PQR, respectively

Q) If AD and PM are medians of triangle ABC and PQR, respectively where Δ ABC ~ Δ PQR, prove that AB / PQ = AD / PM.

Ans:

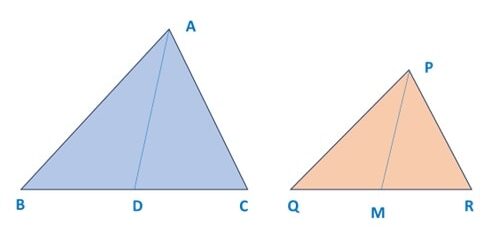
Given that, Δ ABC ~ Δ PQR, therefore

$\frac{AB}{PQ} = \frac{BC}{QR}$

Since AD is median of BC, hence BC = 2BD

Similarly, PM is median of QR, hence QR = 2QM

$\therefore \frac{AB}{PQ} = \frac{2BD}{2QM}$

or $\frac{AB}{PQ} = \frac{BD}{QM}$

and ∠ B = ∠ Q (given that Δ ABC ~ Δ PQR)

$\therefore$ Δ ABD ~ Δ PQM (by SAS similarity)

Therefore, $\frac{AB}{PQ} = \frac{AD}{PM}$

Hence proved.

Contact us