Sides AB and BC and median AD of a triangle ABC are respectively

Q) Sides AB and BC and median AD of a triangle ABC are respectively proportional to sides PQ and QR and median PM of Δ PQR. Show that Δ ABC ~ Δ PQR.

Ans:

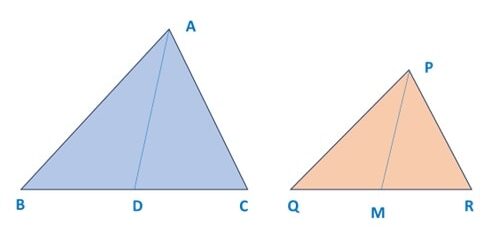
Given that, In Δ ABC and Δ PQR,

$\frac{AB}{PQ} = \frac{BC}{QR} = \frac{AD}{PM}$

Since AD is median of BC, hence BC = 2BD

Similarly, PM is median of QR, hence QR = 2QM

$\therefore \frac{AB}{PQ} = \frac{2BD}{2QM} = \frac{AD}{PM}$

or $\frac{AB}{PQ} = \frac{BD}{QM} = \frac{AD}{PM}$

$\therefore$ Δ ABD ~ Δ PQM

Hence, ∠ B = ∠ Q ……………. (i)

Now In Δ ABC and Δ PQR, we know that,

or $\frac{AB}{PQ} = \frac{BC}{QR}$ (given)

∠ B = ∠ Q from equation (i)

$\therefore$ Δ ABC ~ Δ PQR……….. Hence proved

Contact us