A student noted the number of cars passing through a spot on

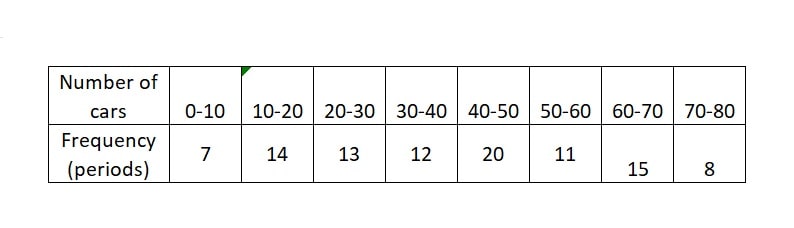
Q) A student noted the number of cars passing through a spot on a road for 100 periods each of 3 minutes and summarised it in the table given below. Find the mean and median of the following data:

Ans:

(i) Let’s re-arrange the data with midpoint of each class, frequency, and multiply midpoint with frequency:

We know that, mean of grouped data is given by:

Mean of grouped data = $\frac{\sum fx}{\sum f} = \frac{4070}{100}$ = 407

Therefore, Mean value of the given data is 407

(ii) To find the median, we need to identify middle value of the data. Let’s rearrange the data.

Step 1: we need to find the cumulative frequency in the frequency table to find the median. Its shown in last column.

Step 2: Next, total number of sub-divisions or Sum of the frequencies = 100. It shown at the bottom of middle column.

Step 3: Next, we need to identify Median Class.

Since the Median class is the class where the cumulative frequency crosses 50% of the half the total number of sub-divisions. Here, 50% of total frequency is 50 and cumulative frequency is crossing is crossing at 66 i.e. at class “40-50”. Hence, our Median class = 40-50

Next, To find the median, we use the formula:

Median = L + [ $(\frac{n}{2} - F)$ x $\frac{h}{f}$ ]